Regular Quadrangular Prism

Definition:

A regular quadrangular prism is a three-dimensional geometric figure with two parallel, congruent square bases and four lateral faces that are rectangles.
This type of prism is also known as a right square prism.

Key Features:

1. Lateral Edge: The lateral edge is the common side shared by two adjacent lateral faces. In a regular quadrangular prism, all lateral edges are
   of equal length and perpendicular to the bases.

2. Height: The height of the prism is the perpendicular distance between the two bases. It is the length of the segment that connects corresponding
   points on the two bases and is perpendicular to both.

3. Diagonal of the Prism: A diagonal of the prism is a line segment that connects two vertices of the bases that do not lie on the same face.
   This diagonal spans across the interior of the prism.

4. Diagonal Plane: A diagonal plane is a plane that passes through a diagonal of the prism and includes the lateral edges. This plane divides
   the prism into two symmetrical parts.

5. Diagonal Section: The diagonal section is the intersection of the prism with a diagonal plane. In a regular quadrangular prism, this section forms
   a rectangle.

6. Perpendicular Section (Orthogonal Section): A perpendicular section is the intersection of the prism with a plane that is perpendicular to its lateral edges.
   This section is typically a square, as it cuts through the prism parallel to the bases.

Additional Information:

• Surface Area: The total surface area of a regular quadrangular prism is the sum of the areas of its two bases and its four lateral faces. The formula
  for the surface area (A) is:

  A=2a²+4ah

  where (a) is the side length of the square base and (h) is the height of the prism.

• Volume: The volume (V) of the prism is given by the product of the area of the base and the height:

  V=a²h

• Properties: All angles between the lateral faces and the bases are right angles. The prism is symmetrical along any plane that passes through its height
  and divides it into two equal halves.

Elements of a regular quadrangular prism

• Base ABCD and A₁B₁C1D1 are equal and parallel to each other
• Lateral faces AA₁D₁D, AA₁B₁B, BB₁C₁C and CC₁D₁D, each of which is a rectangle
• Lateral surface is the sum of the areas of all lateral faces of the prism
• Total surface is the sum of the areas of all bases and lateral faces (the sum of the area of ​​the lateral surface and bases)
• Lateral edges AA₁, BB₁, CC₁ and DD₁.
• Diagonal B₁D
• Base diagonal BD
• Diagonal section BB₁D₁D
• Perpendicular section A₂B₂C₂D₂ .

Properties of a Regular Quadrangular Prism

1. Bases:

   • The bases are two equal squares.
   • The bases are parallel to each other.

2. Lateral Faces:

   • The lateral faces are rectangles.
   • The lateral faces are equal to each other.
   • The lateral faces are perpendicular to the bases.

3. Lateral Edges:

   • The lateral edges are parallel to each other and equal in length.

4. Perpendicular Section:

   • The perpendicular section is perpendicular to all lateral edges and parallel to the bases.
   • The angles of the perpendicular section are right angles.

5. Diagonal Section:

   • The diagonal section of a regular quadrangular prism is a rectangle.

6. Orthogonal Section:

   • The perpendicular (orthogonal) section is parallel to the bases.

7. Additional Properties:

   • The prism has 6 faces, 12 edges, and 8 vertices.
   • The volume of the prism is calculated as the product of the base area and the height:

     V=A_b​⋅h

     , where (A_b) is the area of the base and (h) is the height.
   • The total surface area is the sum of the lateral surface area and twice the base area:

     S=2A_b​+A_l​

     , where (A_l) is the lateral surface area.

Formulas for a regular quadrangular prism

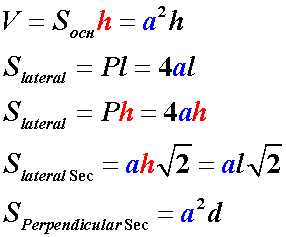
Instructions for solving problems

Problem.

Solution.

The perimeter P_base of the square base is:

P_base = 4a
P_base = 4 times 12
P_base = 48 cm

The lateral surface area A_total is:

 A_total  =P_base x h 
A_total = 48x 14
A_total  = 672 cm²

The total surface area is:

 A_total  = 2A_base + A_lateral 
 A_total = 2 x 144 + 672
A_total = 288 + 672
 A_total  = 960 cm²

Problem

Determine the total surface area of ​​a regular quadrangular prism if its diagonal is 5 cm and the diagonal of the lateral face is 4 cm.

Solution

Let’s denote:

• Side length of the base ( a )
• Height of the prism ( h )

The diagonal of the base can be found using the Pythagorean theorem in the base square:

a² + a² = 5²
2a² = 25
a = √12,5

The diagonal of the lateral face (which is a rectangle) can be expressed as:

h² + 12,5 = 4²
h² + 12,5 = 16
h² = 3,5
h = √3,5

The total surface area will be equal to the sum of the lateral surface area and twice the area of ​​the base.

S = 2a² + 4ah
S = 25 + 4√12,5 * √3,5
S = 25 + 4√43,75
S = 25 + 4√(175/4)
S = 25 + 4√(7*25/4)
S = 25 + 10√7 ≈ 51,46 см² .

Answer: 25 + 10√7 ≈ 51,46 cm² .